If x=√ (3√5) and y=√ (3√5), what is the value of expression x y 2x²y 2xy² x⁴y xy⁴?(x1) (x2) = x 2 3x 2 Thanks for trying but the answer is 459 dududsu2 #3 1 x= 9y xy would then be (9y) y = 10 y^2 9y 10 = 0 y^29y10 = 0 y = 9/2 sqrt41/ 2 (x^3 y^3 = (

If X Y 1 Then Find The Value Of X 3 Y 3 3xy Maths Continuity And Differentiability Meritnation Com
(x+y)3=x3+y3+3xy(x+y)
(x+y)3=x3+y3+3xy(x+y)-(X – Y) 3 = X 3 – Y 3 – 3XY(X – Y) Or (X – Y) 3 = X 3 – Y 3 – 3X 2 Y 3XY 2 Was this answer helpful?It is clear that when $x=y$ we have $x^3y^3=0$ Then use long division to divide $x^3y^3$ by $xy$ and the result will be the equation on the right Another way would be to write $$\left(\frac{x}{y}\right)^3 1$$ Now we wish to find the zeros of this polynomial




If X 3 Y 3 3xy 1 Where X And Y Are To Distinct Real Numbers Then Which Of The Following Can Be Values Of X And Y
So we set it = 0 i*sqrt(3)(x1) = 0 x1 = 0 x = 1 Then substituting that y = y = y = y = 1 So we end up with all solutions {(x,y) x y = 1} plus the one solution (x,y) = (1,1) Now if you were asking about xy instead of xy, the answer would be xy is always either 1 or 2 Are you sure you didn't make a typo and you were asking about xy and not xy?यदि x 2 – 9x 1 = 0 है, तो x 3 1/x 3 का मान क्या है?Mathx=\sqrt {3\sqrt 5}=\sqrt {\dfrac {6–2\sqrt 5}2
In mathematics, the cube of sum of two terms is expressed as the cube of binomial x y It is read as x plus y whole cube It is mainly used in mathematics as a formula for expanding cube of sum of any two terms in their terms ( x y) 3 = x 3 y 3 3 x 2 y 3 x y 2Without doing the maths the answer is very clearly yes!Telangana SCERT Class 9 Math Chapter 2 Polynomials and Factorisation Exercise 25 Math Problems and Solution Here in this Post Telanagana SCERT Class 9 Math Solution Chapter 2 Polynomials and Factorisation Exercise 25
We know that algebraic formula, (x y) 3 = x 3 y 3 3xy (x y) put the value of x y in given equation given, x y = 1 1 = x 3 y 3 3xy X 1 ⇒ x 3 y 3 3xy = 1 Previous Question Next Question Your comments will be displayed only after If x y = 12, and xy = 27, then find the value of x3 y3 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queries 0 Follow 0 A K Daya Sir, added an answer, on 25/9/13 A K Daya Sir answered this x 3 y 3 = (x y) (x 2 xy y 2 ) this formula can be derived from (x y) 3 = x 3 y 3 3xy (x y) x 3 y 3 = (x y) 3 3xy (x y) x 3 y 3 = (x y) (x y) 2 3xy = (x y) x 2 y 2 2xy 3xy = (x y) (x 2 xy y 2 ) Was this answer helpful?




Factorise X X3 Y3 3xy X Y Brainly In



Locus Of All Point P X Y Satisfying X 3 Y 3 3xy 1 Consists Of Union Of Sarthaks Econnect Largest Online Education Community
4 It's two equations, with two variables We can do what we always do You can write y = 8 / x, and substitute into the first equation, obtaining x3 / x3 = 72 From here, we want to write this as a polynomial, so we multiply through x3 so there are no negative powers, and then move everything to the leftThe algebraic identities for class 9 consist of identities of all the algebraic formulas and expressions You must have learned algebra formulas for class 9, which are mathematical rule expressed in symbols but the algebraic identities represent that the equation is true for all the values of the variables For example;Because according to ncert its answer is (polynomial example 23(ii)) if it is wrong then why and where plz me




Factorise X3 9y3 3xy X Y Brainly In




Consider The Function F X Y X 3 Y 3 3xy Chegg Com
35 (1) Upvote (1) Choose An Option That Best Describes Your Problem Answer not in Detail Incomplete Answer Answer Incorrect Others Answer not in Detail Incomplete Answer Answer Incorrect find x^3y^3 The coordinates of point Y are giving The midpoint XY is (3,5) Find the coordinates of point XWe know that (x y) 3 = x 3 y 3 3xy (x y) Using Identity VII ⇒ x 3 y 3 = (x y) 3 3xy (x y) x 3 y 3 = (x y) { (x y) 2 3xy} ⇒ x 3 y 3 = (x y) (x 2 2xy y 2 3ry) Using Identity IV ⇒ x 3 y 3 = (x y) (x 2 xy y 2 ) 350 Views




How To Solve Math X 3 Y 3 Dx 3xy 2dy 0 Quora




The Factors Of X 3 1 Y 3 3x Y Are A X 1 Y X 2 1 Y 2 X Y X Y B X Y 1 X 2 Y 2 1 X Y X Y C X 1 Y X 2 1 Y 2 X Y X Y D 3 X Y 1 X 2 Y 2 1
State reasons for your answer Ans (i) 4x 2 – 3x 7 ⇒ 4x2 – 3x 7x° ∵ All the exponents of x are whole numbers ∴ 4x 2 – 3x 7 is a polynomial in one variable (ii) ∵ All the exponents of yFind an answer to your question prove(xy)^3=x^3y^33xy(xy) mynameiskhan67 mynameiskhan67 Math Secondary School answered Prove (xy)^3=x^3y^33xy(xy) 2 See answers VegotaCorrect answers 1 question Q (999) ^3 =() ^3 =(xy) ^3 = x^3y^33xy(xy) =() ^3= 1000^3 1^() =1, 000,000,() =999, 999,9993, 000,0003, 000 = sir, it is correct or wrong?




If X Y 1 Then Find The Value Of X 3 Y 3 3xy Maths Continuity And Differentiability Meritnation Com
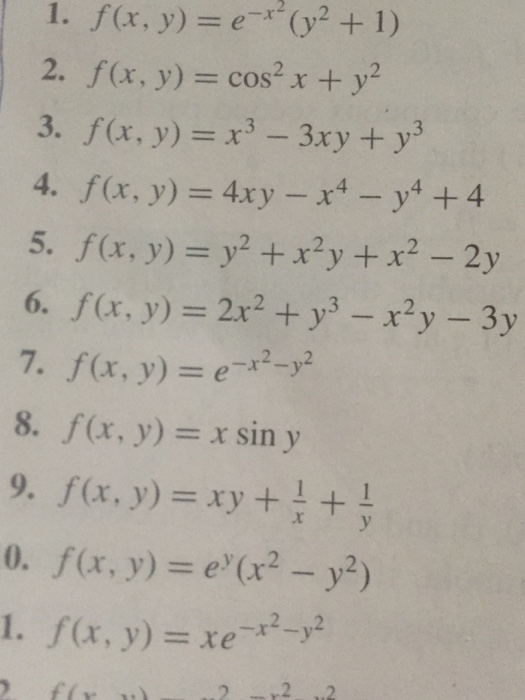



F X Y E X 2 Y 2 1 F X Y Cos 2 X Y 2 Chegg Com
यदि x 2 – 9x 1 = 0 है, तो x 3 1/x 3 का मान क्या है?X a /x b = x ab = 1/x ba;Cube of a binomial can be simplified using the identities \({(x y)}^3 = x^3 y^3 3xy(x y)\)




X X3 Y3 3xy X Y Brainly In




Find All Values Of X Y Where Theorem 3 1 1 The Chegg Com
SOLUTION ⇒ x – y = 3 ⇒ (x – y) 3 = 3 3 ⇒ x 3 – y 3 – 3xy (x – y) = 27 ⇒ x 3 – y 3 – 3xy × 3 = 27 ⇒ x 3 – y 3 – 9xy = 27 Related Questions If x 2 – 9x 1 = 0, then what is the value of x 3 1/x 3? Find an answer to your question if \ x y z = 0 \ then \ show \ that \ {x}^{3} {y}^{3 } {z}^{3} =3xyQuadratic equation, for any given x if ax 2 bx c =0 then x has 2 solutions x=(b√(b 2 4ac)/2a, x=(b√(b 2 4ac)/2a x a y b is not equal to (xy) ab



If X Y 12 And Xy 27 Then Find The Value Of X3 Y3 Polynomials Maths Class 9




X Y 3 X3 Y3 3xy X Y Proof It Maths Polynomials Meritnation Com
(xy)^3 x^3y^33xy(xy) x^3y^3z^33xyz (xyz)(x^2y^2z^2xyyzzx) √ab √a√b √a/b(whole) √a/√b (√a√b)(√a√b) ab (a√b)(a√b) a^2b (√a√b)^2 a2√abb OTHER SETS BY THIS CREATOR GeographyChapter 1Indiasize and location 13 terms ikeepchangingmynameX 0 =1 x a y a = (xy) a, 2 2 3 2 = 6 2; xy3xyxy 来源:本站编辑 主要内容: 本文介绍隐函数√ (x1)√ (y1)=1的定义域、值域、单调性、凸凹性等主要性质,并举例用导数知识求解该函数的单调区间和凸凹区间。 函数的定义域 对隐函数√ (x1)√ (y1)=1有: √ (x1)=1√ (y1)≤1, 不等式




If X 3 Y 3 3xy 1 Where X And Y Are To Distinct Real Numbers Then Which Of The Following Can Be Values Of X And Y




X 3 3x 2 X 3 Factorise Novocom Top
If x y z = 0, show that x3 y3 z3 = 3xyz Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get xy=1 (xy)³=1 x³3x²y3xy²y³=1 3xy(xy)3=1 xy=2/3 (xy)²=1 x²y²2xy=1 x²y²=7/3 (x²y²)(x³y³)=7/3*3=7 x^5x²y³x³y²y^5=7 x^5y^5=x²y²(xy)7=4/97=59/9 已赞 Find an answer to your question if xy=4, then find the value of x^3y^312xy64 please answer someone dhruvrj dhruvrj Math Secondary School answered If xy=4, then find the value of x^3y^312xy64 please answer someone 1 See answer dhruvrj is waiting for your help Add your answer and earn points
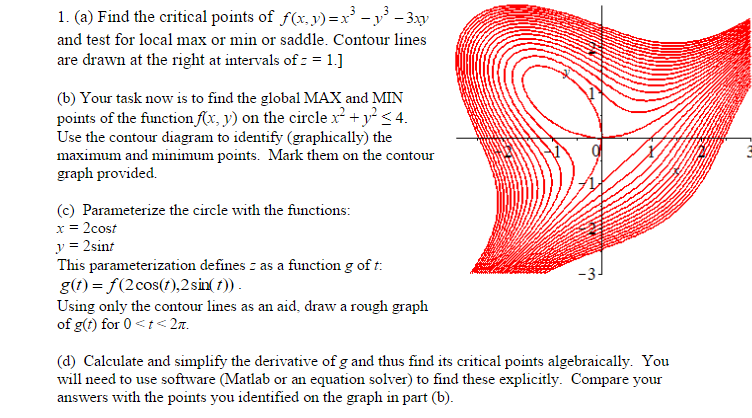



Find The Critical Points Of F X Y X 3 Y 3 3xy Chegg Com
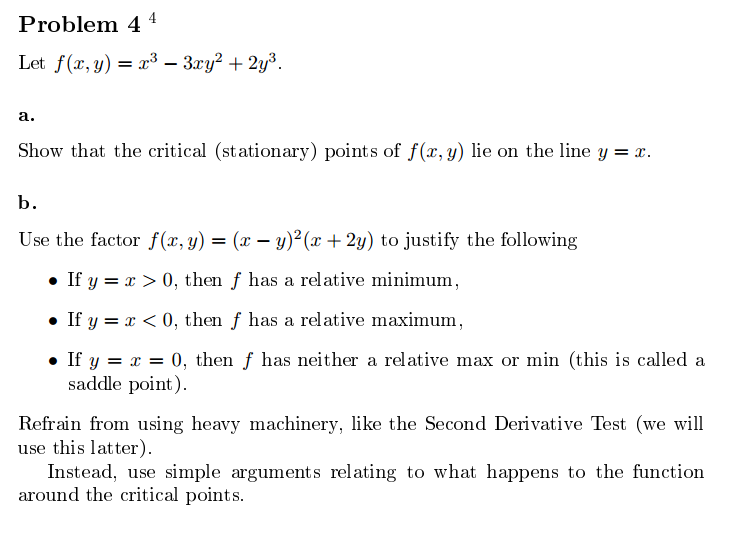



Let F X Y X 3 3xy 2 2y 3 Show That The Chegg Com
1 How do you simplify the cube of a binomial?(xy) 3 = x 3 y 3 3xy(x y) x 2 y 2 = (x y)(x y) x2 = 1/x 2, 24 = 1/16 = 1/2 4 (x a)(x b) = x ab;X^3 y^3 = (x y)(x^2 xy y^2) (identity) (2i) = (2i)(x^2 xy y^2) Divide both sides by 2i x^2 xy y^2 = 1 Add 3xy to both sides x^2 2xy y^2 = 3xy 1 (x y)^2 = 3xy 1 (2i)^2 = 3xy 1 4 = 3xy 1 3xy = 3 xy = 1 y = 1/x, x




X 9y 3xy X Y Answer It Plz With Full Maths Brainly In



Find The Extreme Values Of The Function X 3 Y 3 3xy Sarthaks Econnect Largest Online Education Community
Solution (By Examveda Team) Given, xy = 2 cubing both sides (xy) 3 = 2 3 => x 3 y 3 3xy ( xy) = 8 => x 3 y 3 3×15×2= 8 => x 3 y 3 90 = 8 => x 3 y 3 = 02y=2 y=1 Hence, putting the value of y again in any of the above three equations will give the value of x So, taking equation x=y5 x=1–5 x=4 So the final answers are x=4 and y=1 For any clarification, please update in comments And if you liked the answer do upvote and sorry for any grammatical mistakesThere are two variables and one equation so there will be an infinite number of solutions Let's solve for y xy = xy x = xy y factor out the y x = y (x1) x x − 1 = y so y = x x − 1 x ≠ 1



If X 3 Y 3 9 And X Y 3 Then The Value Of X 4 Y 4 Please Answer Honestly Don T Watch Others Quora




X Y 3 X3 Y3 3xy X Y Proof It Maths Polynomials Meritnation Com
Start your 48hour free trial to unlock this answer and thousands more Enjoy eNotes adfree and cancel anytimeSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreयदि x – y = 3 है, तो x 3 – y 3 – 9xy का मान क्या है?




X X 3 Y 3 3xy X Y




If X 3 Y 3 3xy 2 3x 2y 1 0 Then At 0 1 Dy Dx Youtube
X x 3 y 3 3xy x y Use the distributive property to multiply x y by x 2 x y y 2 and combine like terms If α and β are the zeroes of the polynomial ax bx c find the value of α β write five equivalent fractions to 5 7 how many lines can pass through a given point if p and q are functions of then the equation of the formdy py qydxis equal toaGiven x y = 1, Formula (x y) 3 = x 3 y 3 3xy (x y) Calculations According to the question (x y) 3 = x 3 y 3 3xy (x y) ⇒ 1 3 = x 3 y 3 3xy × 1 ⇒ x 3 y 3 3xy – 1 = 1 – 1 ∴ x 3 y 3 3xy – 1 = 0 Download Question With Solution PDF ››Here given are, XY=7, let's say it is formula 1 in order to make it kinda convenient and easy to understand And XY=10, let's mark it as 2 Now, see formula 1 can be also written as,



X Y 3 X Y 3 Simplify




If X 3 3xy 2y 3 17 Then In Terms Of X And Y Chegg Com
(i) (99) 3 (ii) (102) 3 (iii) (998) 3 Solution We will use the following algebraic Identities to evaluate the given expressions (x y) 3 = x 3 y 3 3xy(x y) (x y) 3 = x 3 y 3 3xy(x y) (i) (99) 3 = (100 1) 3 Identity Here we can use (x y) 3 = x 3 y 3 3xy(x y) Let us substitute x = 100, y = 1 (99) 3 = (100) 3 (1) 3 3(100)(1)(100 1)Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more1 Which of the following expressions are polynomials in one variable and which are not?




Prove That X2 Y2 C X2 Y2 2 Is The General Solution Of The Differential Equation X3 3xy2 Dx Y3 3x2y Dy Where C Is Parameter Mathematics Shaalaa Com
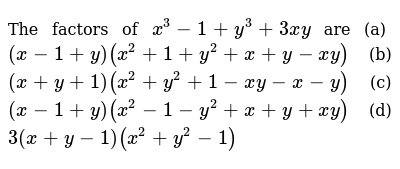



The Factors Of X 3 1 Y 3 3x Y Are A X 1 Y X 2 1 Y 2 X Y X Y B X Y 1 X 2 Y 2 1 X Y X Y C X 1 Y X 2 1 Y 2 X Y X Y D 3 X Y 1 X 2 Y 2 1
We know that ( x y ) 3 = x 3 y 3 3xy ( x y ) Now subtracting 3xy ( x y ) on both sides we get ( x y ) 3 3xy ( x y ) = x 3 y 3 3xy ( x y ) 3xyNear (x, y)=(3245, 197), (309, 845), (25, 9) and (2365, 15) And since the two equations can be combined into a quartic in x, (or a quartic in y) and a quartic has 4 roots in the complex numbers and we have found 4 real roots then these are the only solutions even if you allowed x and y to be complex numbers Here, exponent of every variable is a whole number, but x 10 y 3 t 50 is a polynomial in x, y and t, ie, in three variables So, it is not a polynomial in one variable Ex 21 Class 9 Maths Question 2




Factories X3 9y3 3xy X Y Brainly In
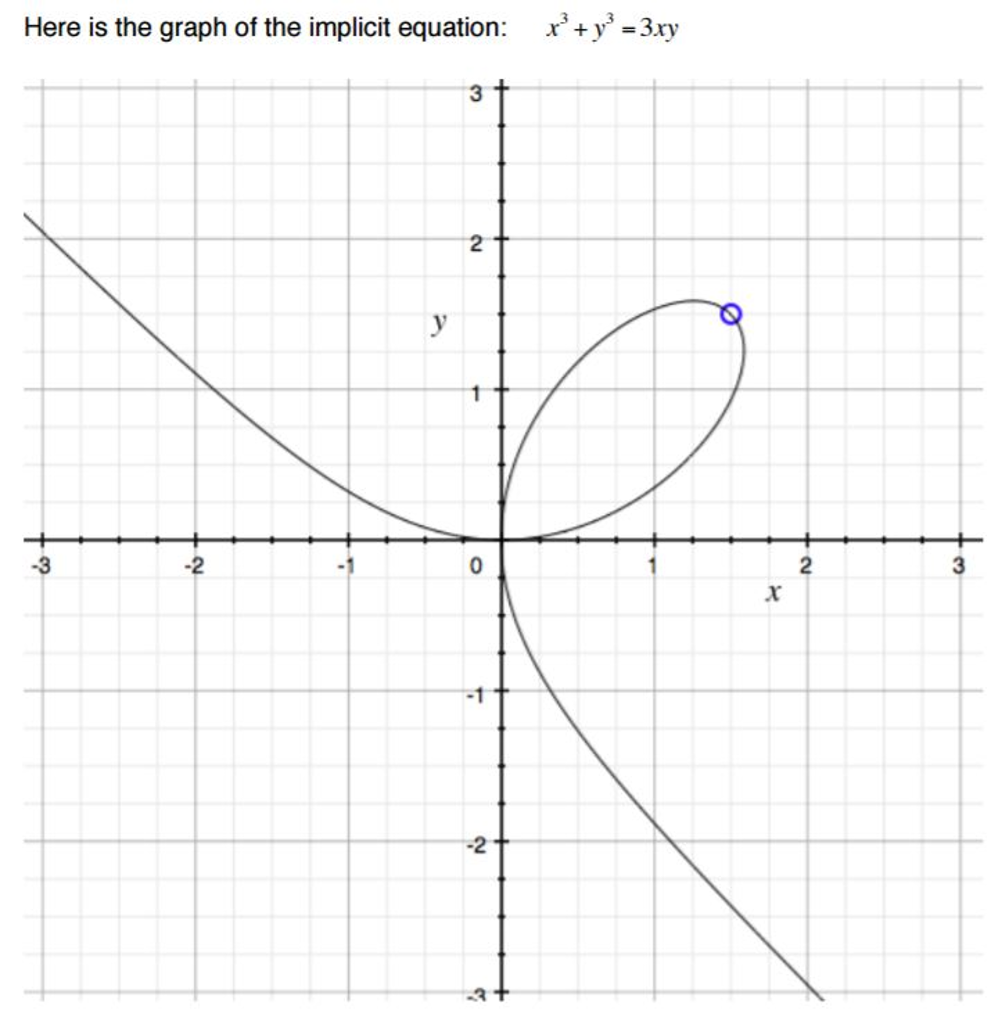



Here Is The Graph Of The Implicit Equation X 3 Y 3 Chegg Com
• (x y z) 2 = x 2 y 2 z 2 2xy 2yz 2zx • (x y) 3 = x 3 y 3 3xy(x y) • (x – y) 3 = x 3 – y 3 – 3xy(x – y) • x 3 y 3 z 3 – 3xyz = (x y z)(x 2 y 2 z 2 – xy – yz – zx) Also, check the NCERT Solutions for Class 9 Maths Chapter 2 from the following link Also Check NCERT Books for Class 9Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyIf x 2 – 9x 1 = 0, then what is the value of x 3 1/x 3?




Verify X Y 3 X3 Y3 3xy X Y Brainly In




Ppt Polynomials Powerpoint Presentation Free Download Id
(x y)^3 = (x^3) (y^3) 3xy(x y) so, (3)^3 = 9 3xy(3) so, 27 = 9 9xy so, (27 9)/9 = xy so, xy = 18/9 = 2




Analyze The Product Of X 3 9y 3 3xy X Y
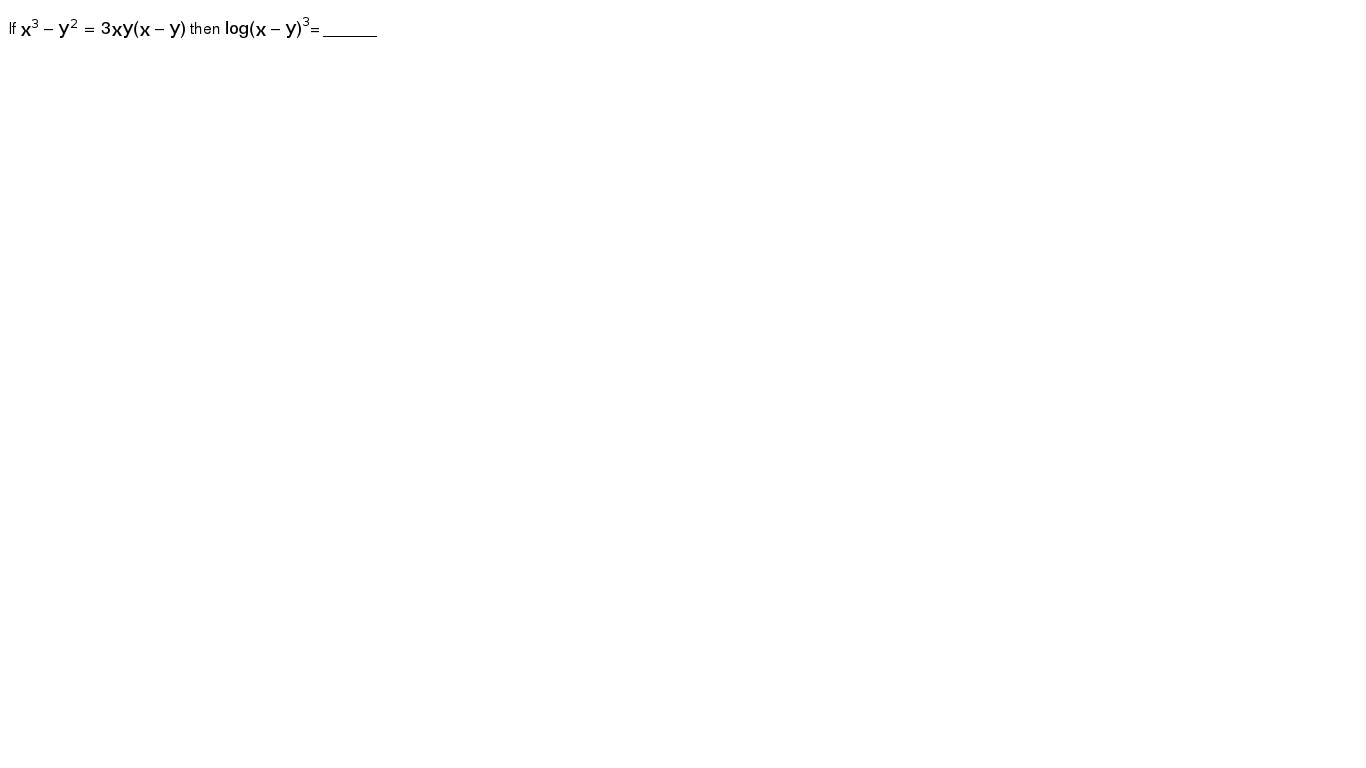



If X 3 Y 2 3xy X Y Then Log X Y 3



X 3 Y 3 3xy 2 Find Dy Dx Using Implicit Differentiation Youtube



The Two Curves X 3 3xy 2 2 0 And 3x 2y Y 3 2 0 Intersect At An Angle Of Sarthaks Econnect Largest Online Education Community




Second Partials Test For F X Y X 3 3xy Y 3 Youtube



Solve Dy Dx 3xy Xy 3 30 Dy Dx X 2y 3 2x Chegg Com




X X3 Y3 3xy X Y Maths Polynomials Meritnation Com




Find The Local Extrema Of F X Y X 3 3xy Y 3 Chegg Com



What Is The Solution To This Homogeneous Differential Equation Dy Dx Y 3 3x 2y X 3 3xy 2 Quora




Transform X 3 Y 3 3xy 0 Mathematics Stack Exchange




How To Verify Algebric Identity X Y 3 X3 3xy X Y Y3 Maths Polynomials Meritnation Com
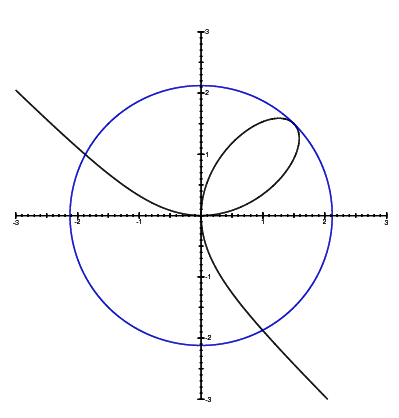



Relplot Equation Plotter




How To Evaluate Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube




Proof Of Formulae I X Y 3 X 3 Y 3 3xy X Y Ii X 3



Maximum Value Of The Function F X Y X 3 Y 3 3xy Is Sarthaks Econnect Largest Online Education Community



Rut Gọn Phan Thức X Y 3 3xy X Y Y 3 X 6y Vietjack Com




If X 3 Y 3 1 3xy Where X Y Determine The Value Of X Y 1
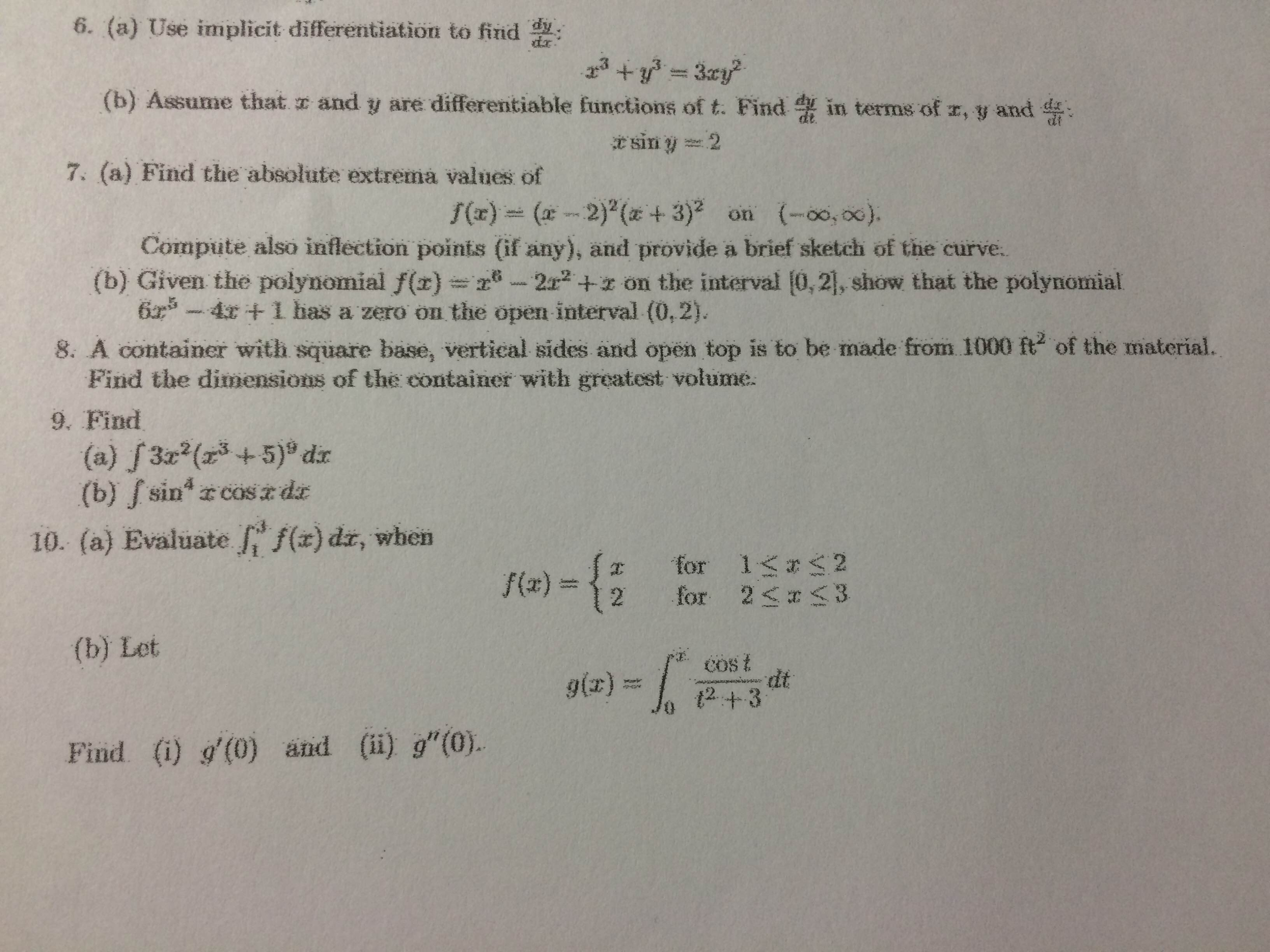



6 A Use Implicit Differentiation To Find Dy Dx Chegg Com




The Sign Of Y 3 3xy X 3 Descartes Folium Download Scientific Diagram




Cho X Y 1 Chứng Minh X 3 3xy Y 3 1 Toan Học Lớp 8 Bai Tập Toan Học Lớp 8 Giải Bai Tập Toan Học Lớp 8 Lazi Vn Cộng đồng Tri Thức Giao Dục




Let F X Y X 3 3x 2y 3 2y 2 We Find Fx X Y 3x 2 Chegg Com




X Y Z 0 Show That X3 Y3 Z3 3xyz Novocom Top



Solve The Differential Equation Dy Dx X 3 Y 3 3xy 2 Sarthaks Econnect Largest Online Education Community




X Y 3 X 3 Y 3 3xy X Y Verify Maths Meritnation Com



1
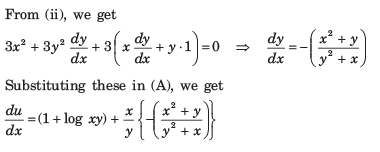



If U X Log Xy Wherex3 Y3 3xy 1 Then Du Dx Is Equal Toa B C D Correct Answer Is Option A Can You Explain This Answer Edurev Electronics And Communication Engineering Ece




Tim Nghiệm Nguyen Dương X 3 Y 3 3xy 1 Toan Học Lớp 9 Bai Tập Toan Học Lớp 9 Giải Bai Tập Toan Học Lớp 9 Lazi Vn Cộng đồng Tri Thức Giao Dục




X Y 3 X3 Y3 3xy X Y Brainly In



Factorize X X 3 Y 3 3xy X Y Sarthaks Econnect Largest Online Education Community




X 3 3xy 2 Dx Y 3 3x 2y Dy Youtube




How To Know At A Glance That Math X 3 3xy 2 2y 3 Math Is Factorable Quora




If X 3 Y 2 3xy X Y Then Log X Y 3



Www Slu Se Contentassets 4e2242ba2b5d4f5bc1dda8ec Facitstud2 Pdf




Factorise X X 3 3 3x 2y X Y Brainly In
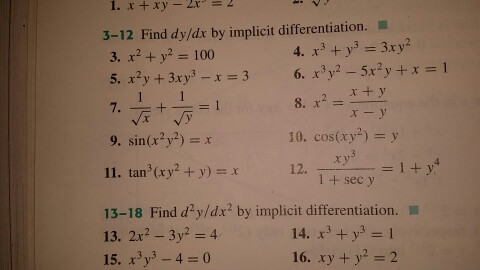



Find Dy Dx By Implicit Differentiation X 2 Y 2 Chegg Com
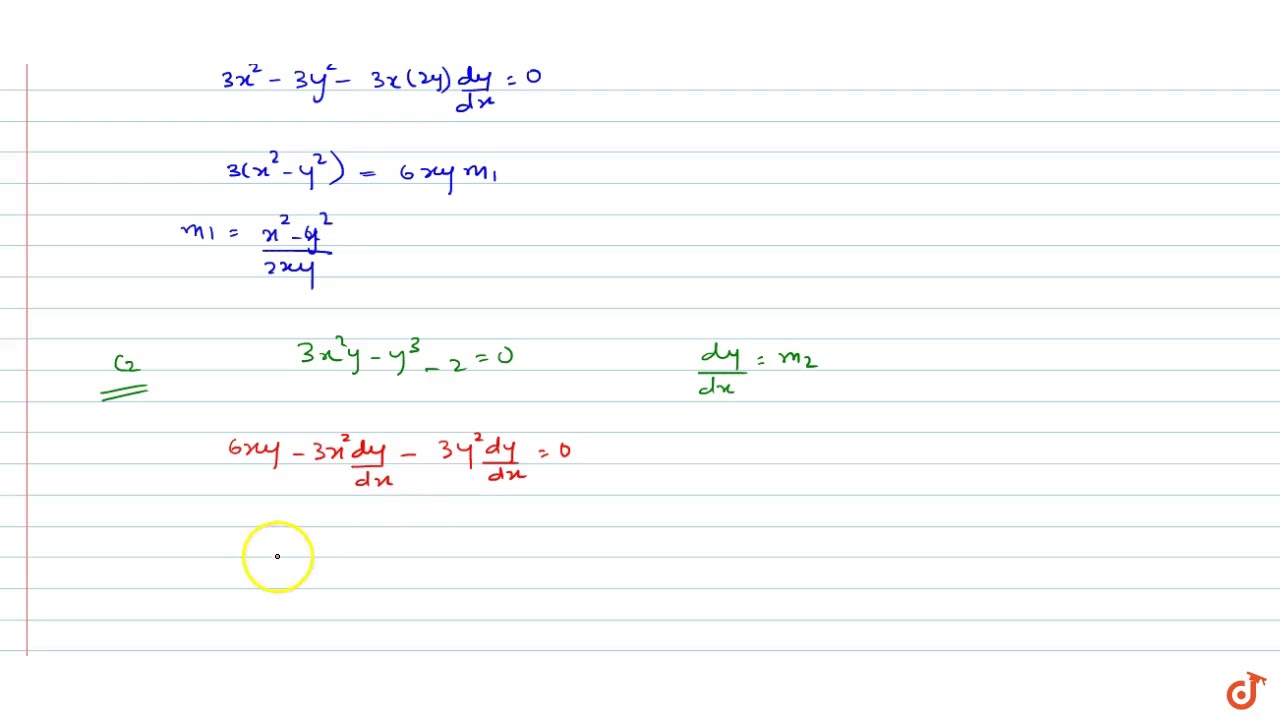



The Two Curves X 3 3xy 2 2 0 And 3x 2y Y 3 2 0 Youtube



If X Y 2 Then What Is The Value Of X Y 6xy Quora




Ex 12 2 4 A What Should Be Added To X 2 Xy Y 2 To Obtain




न म न व य जक क ग णनखण ड क ज य X X 3 Y 3 3xy X Y Youtube




X 3 Y 3 Z 3 Novocom Top
.png)



X Y 3 X3 Y3 Novocom Top



If Math X 3 Y 3 3xy 1 Math What Is The Minimum Value Of Math X 2 Y 2 Math Quora




Giải Toan Tren Mạng Giup Toi Giải Toan Hỏi đap Thảo Luận Về Toan Học Học Trực Tuyến Olm
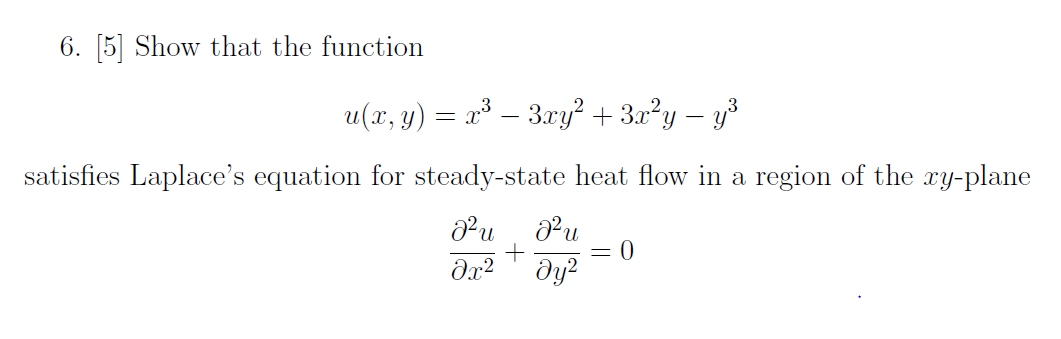



Show That The Function U X Y X 3 3xy 2 3x 2y Chegg Com




Factorise X3 9y3 3xy X Y Brainly In




Solve Differential Equation X 3 3xy 2 Dx Y 3 3x 2y Dy Maths Differential Equations Meritnation Com



Polynomials



Search Q X Y 5e3 Simplify Tbm Isch
.png)



X X3 Y3 3xy X Y Maths Polynomials Meritnation Com




Which Of The Following Is A Factor Of X Y 3 X 3 Y 3



1




If X Y 7 And X3 Y3 133 Find I Xyii X Y Brainly In




The Number Of Critical Points Of F X Y X 3 Chegg Com




Prove X Y 3 X 3 Y 3 3xy X Y Brainly In




Solve X 3 2y 3 Dx 3xy 2dy 0




If X 3 Y 2 3xy X Y Then Log X Y 3



How To Draw The Graph Of X 3 Y 3 3xy Quora
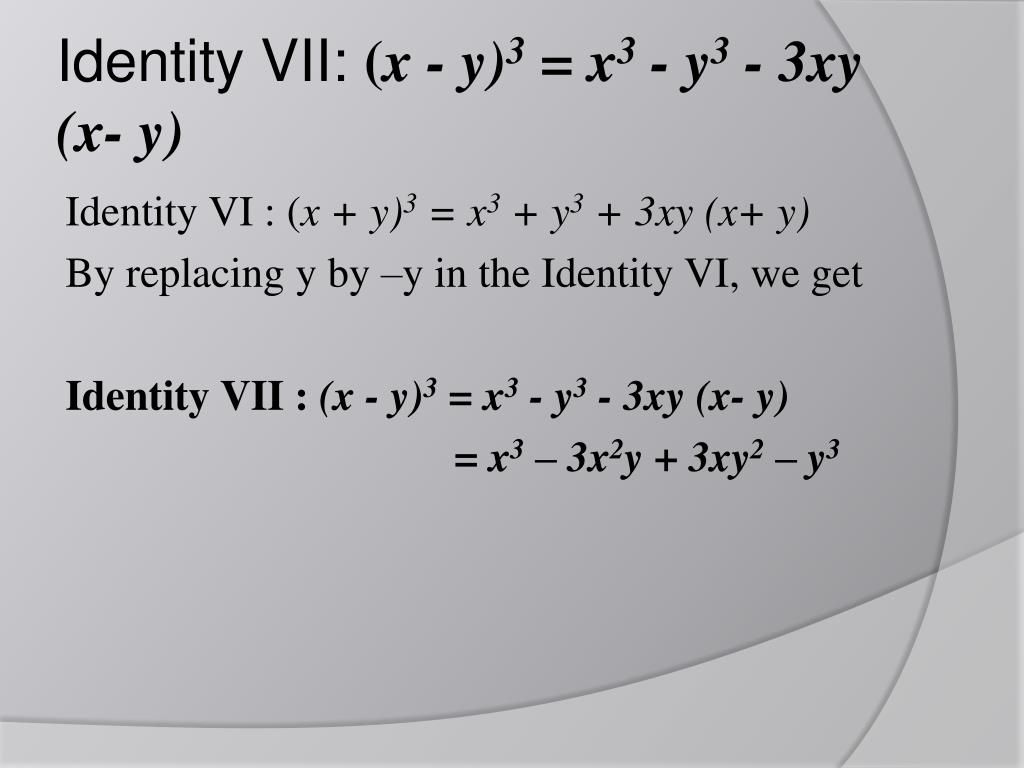



Ppt Polynomials Powerpoint Presentation Free Download Id




Cau Hỏi Của Huỳnh Ngọc Nhien Toan Lớp 8 Học Trực Tuyến Olm




Cho X Y 1 Tinh Gia Trị Của Biểu Thức X 3 Y 3 3xy Cho X Y 1 Tinh Gia Trị Của Biểu Thức X 3 Y 3




Tinh Gia Trị Biểu Thức A X 3 3xy X Y Y 3 X 2 2xy Y 2 Khi X Y 7 Cau Hỏi Hoidap247 Com
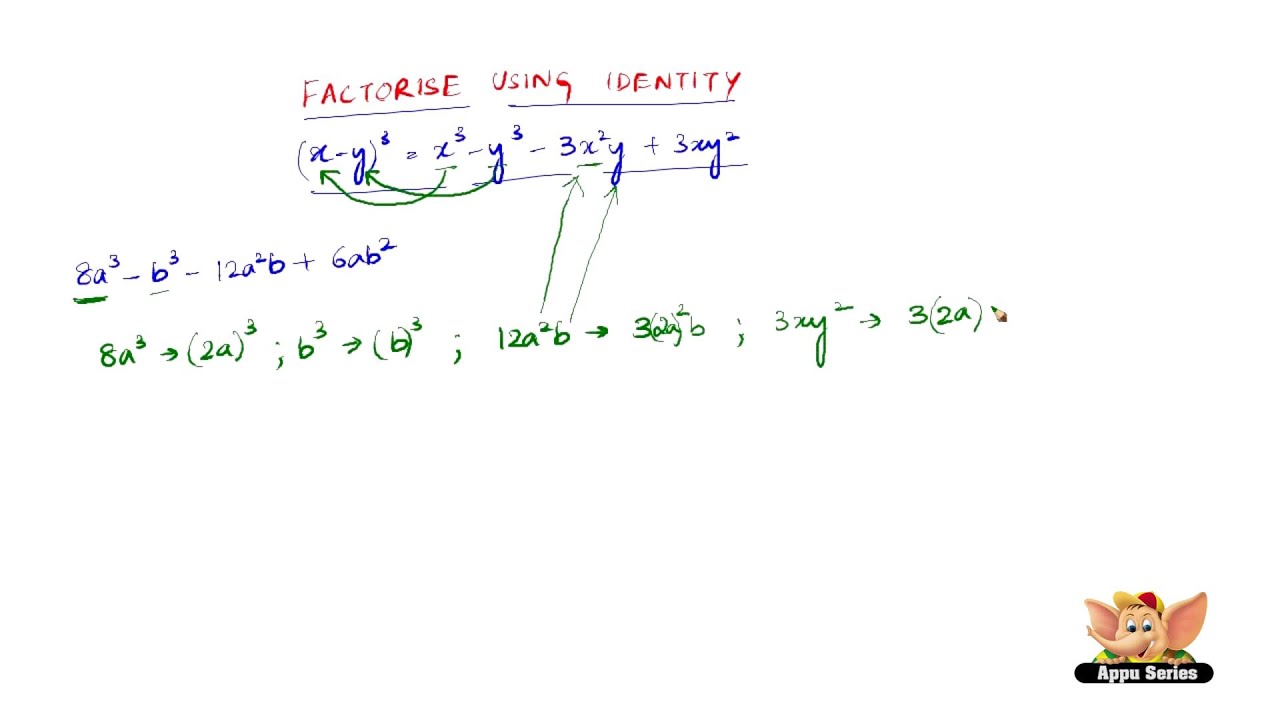



How To Factorise Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube




Factorise X3 9y3 3xy X Y Brainly In




X Y 3 X3 Y3 3xy X Y Brainly In
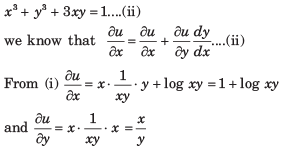



If U X Log Xy Wherex3 Y3 3xy 1 Then Du Dx Is Equal Toa B C D Correct Answer Is Option A Can You Explain This Answer Edurev Electronics And Communication Engineering Ece




Expand X X Y 3 3xy X Y
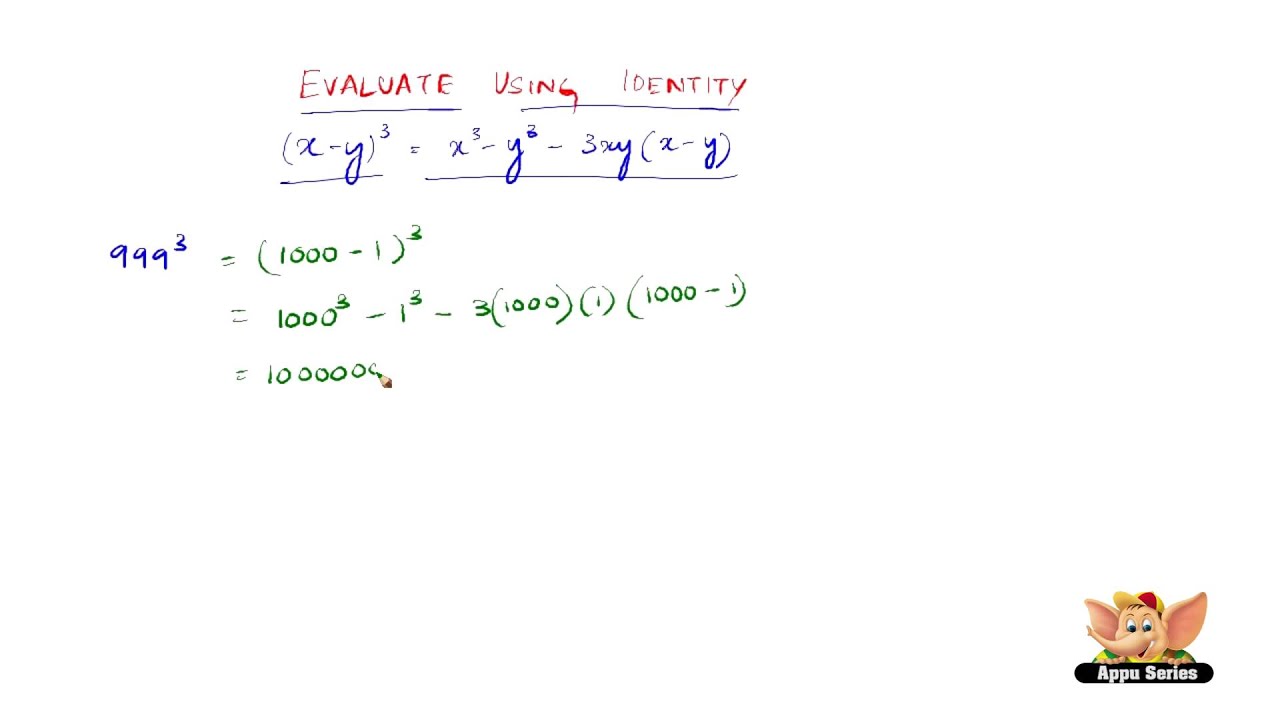



How To Evaluate Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube




X3 3x2 Y 3xy2 Y3 X3 See How To Solve It At Qanda




X 9y 3xy X Y Answer It Plz With Full Maths Brainly In




Factorise X Y 3 X 3 Y 3 3xy X Y Brainly In




The Curves X 3 3xy 2 A And 3x 2 Y Y 3 B Where A And B Are Constants Cut Each Other At An Angle Of




K 6y Y 1 7y 7y Jndei M 3x 4x N 6x X Je Gauthmath




If X Y 1 Then Find The Value Of X3 Y3 3xy Brainly In




If X 3 3xy 2 14 Y 3 3yx 2 13 And X Y Are Real Find The Maths Polynomials Meritnation Com



0 件のコメント:
コメントを投稿